Important update from TheSchoolRun
For the past 13 years, TheSchoolRun has been run by a small team of mums working from home, dedicated to providing quality educational resources to primary school parents. Unfortunately, rising supplier costs and falling revenue have made it impossible for us to continue operating, and we’ve had to make the difficult decision to close. The good news: We’ve arranged for another educational provider to take over many of our resources. These will be hosted on a new portal, where the content will be updated and expanded to support your child’s learning.
What this means for subscribers:
- Your subscription is still active, and for now, you can keep using the website as normal — just log in with your usual details to access all our articles and resources*.
- In a few months, all resources will move to the new portal. You’ll continue to have access there until your subscription ends. We’ll send you full details nearer the time.
- As a thank you for your support, we’ll also be sending you 16 primary school eBooks (worth £108.84) to download and keep.
A few changes to be aware of:
- The Learning Journey weekly email has ended, but your child’s plan will still be updated on your dashboard each Monday. Just log in to see the recommended worksheets.
- The 11+ weekly emails have now ended. We sent you all the remaining emails in the series at the end of March — please check your inbox (and spam folder) if you haven’t seen them. You can also follow the full programme here: 11+ Learning Journey.
If you have any questions, please contact us at [email protected]. Thank you for being part of our journey it’s been a privilege to support your family’s learning.
*If you need to reset your password, it will still work as usual. Please check your spam folder if the reset email doesn’t appear in your inbox.
A teacher's guide to adding fractions
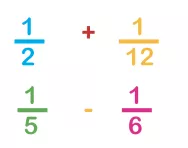
Adding and subtracting fractions in Key Stage 1
In Year 1, children begin their journey by understanding fractions as equal parts of a whole or a set. While addition and subtraction of fractions may not be explicitly taught, children can engage in practical activities to develop a foundation for future learning. They can divide objects into equal halves or quarters, count the number of shaded and unshaded parts and compare the fractions based on their sizes.
As children move up into Year 2, they may continue to explore fractions as equal parts of a whole or a set. Addition and subtraction of fractions are introduced using visual representations, such as shapes or diagrams. Here's a breakdown of the process:
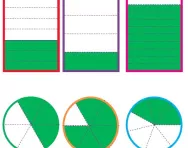
- Addition: Children are taught to add fractions by counting and combining the shaded parts. For example, to add two quarters and one quarter, they can count the total number of shaded parts (2 + 1 = 3) and express it as a fraction over the common denominator (3/4).
- Subtraction: Children may also learn that they can subtract fractions by removing or counting the shaded parts from the whole. For instance, to subtract one half from three quarters, they can visually see that there is one shaded part remaining (3 - 2 = 1). The result is expressed as a fraction over the common denominator (1/4).

Adding and subtracting fractions in lower Key Stage 2
As children move up into Year 3, they will start comparing fractions and adding/subtracting fractions with the same denominator. Here's a breakdown:
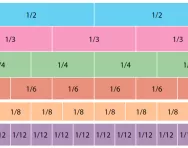
- Addition: When adding fractions with the same denominator, children simply add the numerators while keeping the denominator unchanged. For example, to add 2/5 and 3/5, they add the numerators (2 + 3 = 5) and retain the common denominator (5/5), which simplifies to 1 whole.
- Subtraction: Subtraction of fractions with the same denominator follows a similar process. Children just have to subtract the numerators while keeping the denominator unchanged. For instance, to subtract 4/6 from 5/6, they subtract the numerators (5 - 4 = 1) and keep the common denominator (1/6).
In Year 4, children deepen their understanding of fractions and begin adding/subtracting fractions with different denominators. Here is how to tackle this:
- Addition: When adding fractions with different denominators, children find a common denominator. They can either identify the least common multiple (LCM) of the denominators or multiply the denominators together. Once they have a common denominator, they adjust the numerators accordingly and add them. For example, to add 1/4 and 1/3, they find the common denominator of 12 (LCM of 4 and 3), adjust the fractions to have the common denominator (3/12 + 4/12 = 7/12). It is important to times the numerator by the same amount e.g. in this example, 4 has to be multiplied by 3 to make the denominator of 12 and so the numerator needs to be multiplied by this (1 x 3 = 3).
- Subtraction: Subtraction of fractions with different denominators follows the same process as addition. Children must find a common denominator, adjust the fractions, and subtract the numerators. For instance, to subtract 2/5 from 3/8, they find the common denominator of 40 (8 multiplied by 5), adjust the fractions (15/40 - 16/40 = -1/40), and simplify if necessary.
Adding and subtracting fractions in upper Key Stage 2
As children progress up into the final years of their primary education, they must consolidate their understanding of adding and subtracting fractions, including mixed numbers and improper fractions. Here is a step by step guide for adding fractions when the numbers might be mixed or there are improper fractions:
Step 1: Find a common denominator
Identify the denominators of the fractions you are adding.
Determine the least common multiple (LCM) of the denominators. This will be the smallest number that both denominators divide evenly into.
Use the LCM as the common denominator for the fractions.
Step 2: Adjust the fractions
Multiply the numerator and denominator of each fraction by the same value to make their denominators equal to the common denominator found in Step 1.
This adjustment does not change the value of the fraction.
Step 3: Add the numerators
Add the numerators of the adjusted fractions while keeping the common denominator the same.
Write the sum of the numerators over the common denominator.
Step 4: Simplify the fraction (if necessary)
If possible, simplify the fraction by finding the greatest common divisor (GCD) of the numerator and denominator and dividing both by it.
Simplifying the fraction will give the answer in its simplest form.
Here is an example:
Let's add 3/8 and 5/12:
- Step 1: Find a common denominator: The denominators are 8 and 12. The LCM of 8 and 12 is 24.
- Step 2: Adjust the fractions: 3/8 can be multiplied by 3/3 to have a denominator of 24. 5/12 can be multiplied by 2/2 to have a denominator of 24.
- Step 3: Add the numerators: (3 × 3) + (5 × 2) = 9 + 10 = 19. Write the sum of the numerators over the common denominator: 19/24.
- Step 4: Simplify the fraction (if necessary): 19/24 is already in its simplest form.
The process for subtracting fractions is very similar. Let’s give it a go:
Step 1: Find a common denominator
Identify the denominators of the fractions involved in the subtraction.
Determine the least common multiple (LCM) of the denominators. This will be the smallest number that both denominators divide evenly into.
Use the LCM as the common denominator for the fractions.
Step 2: Adjust the fractions
Multiply the numerator and denominator of each fraction by the same value to make their denominators equal to the common denominator found in Step 1.
This adjustment does not change the value of the fraction.
Step 3: Subtract the numerators
Subtract the numerators of the adjusted fractions while keeping the common denominator the same.
Write the difference of the numerators over the common denominator.
Step 4: Simplify the fraction (if necessary)
If possible, simplify the fraction by finding the greatest common divisor (GCD) of the numerator and denominator and dividing both by it.
Simplifying the fraction will give the answer in its simplest form.
Example of Subtraction: Let's subtract 2/3 from 5/6
- Step 1: Find a common denominator: The denominators are 3 and 6. The LCM of 3 and 6 is 6.
- Step 2: Adjust the fractions: 5/6 does not need any adjustment since its denominator is already 6. 2/3 can be multiplied by 2 to give it a denominator of 6 and a numerator of 4.
- Step 3: Subtract the numerators: (5 - 2) = 3. Write the difference of the numerators over the common denominator: 3/6.
- Step 4: Simplify the fraction (if necessary): The fraction 3/6 can be simplified by finding the greatest common divisor (GCD) of the numerator and denominator, which is 3. Dividing both the numerator and denominator by 3 gives us 1/2.
- Therefore, the subtraction of 2/3 from 5/6 is equal to 1/2.