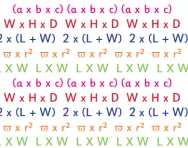Important update from TheSchoolRun
For the past 13 years, TheSchoolRun has been run by a small team of mums working from home, dedicated to providing quality educational resources to primary school parents. Unfortunately, rising supplier costs and falling revenue have made it impossible for us to continue operating, and we’ve had to make the difficult decision to close. The good news: We’ve arranged for another educational provider to take over many of our resources. These will be hosted on a new portal, where the content will be updated and expanded to support your child’s learning.
What this means for subscribers:
- Your subscription is still active, and for now, you can keep using the website as normal — just log in with your usual details to access all our articles and resources*.
- In a few months, all resources will move to the new portal. You’ll continue to have access there until your subscription ends. We’ll send you full details nearer the time.
- As a thank you for your support, we’ll also be sending you 16 primary school eBooks (worth £108.84) to download and keep.
A few changes to be aware of:
- The Learning Journey weekly email has ended, but your child’s plan will still be updated on your dashboard each Monday. Just log in to see the recommended worksheets.
- The 11+ weekly emails have now ended. We sent you all the remaining emails in the series at the end of March — please check your inbox (and spam folder) if you haven’t seen them. You can also follow the full programme here: 11+ Learning Journey.
If you have any questions, please contact us at [email protected]. Thank you for being part of our journey it’s been a privilege to support your family’s learning.
*If you need to reset your password, it will still work as usual. Please check your spam folder if the reset email doesn’t appear in your inbox.
What are the commutative, associative and distributive property?

What are the commutative, associative and distributive property?
The commutative, associative and distributive property are used in algebra to help us solve number problems.
The commutative property explained for parents
The word 'commutative' comes from 'commute' or 'move around', so the commutative property refers to being able to move numbers around within number sentences.
For example: 2 + 3 gives the answer 5, and if we move the numbers around to make 3 + 2, we still get the answer 5. Equally, with multiplication: 6 x 4 = 24, just as 4 x 6 = 24.
In algebra we would write the commutative property as:
- a + b = b + a
- a × b = b × a
The commutative property applies to addition and multiplication, but not to subtraction or division.
Primary-school children are extremely unlikely to hear the words 'commutative property', but children in Year 2 will be taught that addition of numbers can be done in any order, but subtraction of numbers cannot.
The associative property explained for parents
The associative property says that when we add or multiply numbers it doesn't matter how we group them.
This rule applies to numbers that are grouped within brackets, for example: 2 + (3 + 4) or 5 x (2 x 3).
We put calculations in brackets when we want someone to do that particular calculation first. However, when it comes to a group of numbers that need to be added, it does not matter where the brackets go, the answer will still be the same. Equally, with a group of numbers that need to be multiplied, the answer will be the same regardless of how the numbers are grouped.
In algebra we write the associative property as:
- (a + b) + c = a + (b + c)
- (a × b) × c = a × (b × c)
The associative property applies to addition and multiplication, but not to subtraction or division.
It is unlikely that children will hear the term 'associative property' at primary school, but they may be asked to solve a problem, such as the following, where the rule is applied:
Three friends have collected pine cones and are collecting them in a container. Mary and Paul each collect 20 pine cones. John adds another 15 to the collection. How many are there altogether?
When solving the word problem above, (20 + 20) + 15 = 20 + (20 + 15)
The distributive property explained for parents
This is the rule that says that multiplication distributes over addition.
For example: if we want to carry out the following multiplication 2(5 +3), it means that we are working out what 2 lots of 5 are, plus 2 lots of 3, so the 2x can be 'distributed' across the 5 + 3, into 2 x 5 and 2 x 3. This can be written as the following formula:
- a × (b + c) = a × b + a × c
Again, the term 'distributive property' will not be explicitly referred to at primary school, however, children may be asked to solve a problem similar to the following:
There are 25 children in Birch Class and 28 children in Chestnut Class. Each child in both classes needs to bring a pair of gloves to school on Monday. How many individual gloves will be brought in altogether?
Here, the child would need to realise that they can either work this out as:
- 25 + 28 = 53
- 2 x 53 = 106
Or they could do:
- 2 x 25 = 50
- 2 x 28 = 56
- 50 + 56 = 106
How algebra is taught in KS2
Children will start to learn algebra in Year 6, where they will learn about how to use simple formulae and find missing numbers in equations.
It is not stated in the national curriculum that KS2 children need to learn about the use of brackets in calculations. It is possible, however, that more able Year 6 children will be introduced to the use of brackets; the rules about the order in which to complete calculations are taught as BODMAS (Brackets, Other / Indices, Division, Multiplication, Addition and Subtraction).