✕

Important update from TheSchoolRun

For the past 13 years, TheSchoolRun has been run by a small team of mums working from home, dedicated to providing quality educational resources to primary school parents. Unfortunately, rising supplier costs and falling revenue have made it impossible for us to continue operating, and we’ve had to make the difficult decision to close. The good news: We’ve arranged for another educational provider to take over many of our resources. These will be hosted on a new portal, where the content will be updated and expanded to support your child’s learning.

What this means for subscribers:

  • Your subscription is still active, and for now, you can keep using the website as normal — just log in with your usual details to access all our articles and resources*.
  • In a few months, all resources will move to the new portal. You’ll continue to have access there until your subscription ends. We’ll send you full details nearer the time.
  • As a thank you for your support, we’ll also be sending you 16 primary school eBooks (worth £108.84) to download and keep.

A few changes to be aware of:

  • The Learning Journey weekly email has ended, but your child’s plan will still be updated on your dashboard each Monday. Just log in to see the recommended worksheets.
  • The 11+ weekly emails have now ended. We sent you all the remaining emails in the series at the end of March — please check your inbox (and spam folder) if you haven’t seen them. You can also follow the full programme here: 11+ Learning Journey.

If you have any questions, please contact us at [email protected]. Thank you for being part of our journey it’s been a privilege to support your family’s learning.

*If you need to reset your password, it will still work as usual. Please check your spam folder if the reset email doesn’t appear in your inbox.

What is a percentage?

What is a percentage?
We explain what a percentage is, how children are taught to understand the concept, to find percentages of numbers and to compare fractions of amounts to percentages of amounts.

What is a percentage?


A percentage is a number or ratio expressed as a fraction of 100. When we talk about percentages, we imagine that 'a whole' has been divided into 100 equal parts. 

Percentages in primary-school

Percentages are best understood in practical terms. For example:

If a park covers 5km² and 1km² is covered in grass, we know that 1/5 of the park is covered in grass. If we convert this to a percentage, we say that 20% of the park is covered in grass, because 20/100 is equivalent to 1/5.

If there are 30 pupils in a class and 15 of them have packed lunches rather than school dinners, we can say that 1/2 the class have packed lunches. If we convert this to a percentage, we say that 50% of the class have packed lunches, because 50/100 is equivalent to 1/2.
 

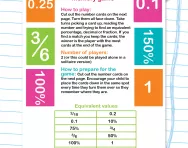
Children are introduced to decimals in Year 4 where they are taught to recognise the equivalence between decimal and fraction forms. They need to know that 0.5 is equivalent to 1/2, 0.25 is equivalent to 1/4, 0.01 is equivalent to 1/100 and 0.1 is equivalent to 1/10.

This knowledge gives them a good grounding for learning about the concept of percentages which is introduced in Year 5.

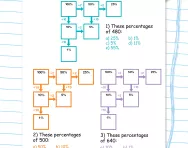
Finding percentages of numbers

In Year 5, children learn to recognise the percent symbol (%) and understand that per cent relates to 'number of parts per hundred'. They need to write percentages as a fraction with denominator 100 and also as a decimal. For example: they might be shown 20% and asked to write this as 20/100 and 0.2.

In Year 6, children move onto finding percentages of amounts:

For example, they may be asked to find: 10% of 4kg

Here, they would need to know that because 10 is 1/10 of 100, they are basically finding 1/10 of the quantity. To find 1/10 of something you need to divide it by 10. So they would need to divide 4kg (or 4000g) by 10 to make 400g.

They may also be asked to find: 5% of £5

Here, they would need to first find 10% of £5. The best way to do this would be to divide 500p by 10, which equals 50p. Since they are finding 5% which is half of 10%, they would need to halve the 50p which would give them 25p.

They may also be asked to find: 15% of 8m

Here they would need to find 10% of 8m (800cm ÷ 10 = 80cm) and 5% of 8m (80cm ÷ 2 = 40cm) and then add them together (80cm + 40cm) to make 120cm.

They may also be asked to find: 40% of £15

Here they would need to find 10% of £15 (1500p ÷ 10 = 150p) and then multiply this by 4 (150p x 4 = 600p = £6).

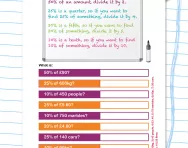
Percentage word problems

Often, children will need to carry out problem-solving related to percentages. They may be asked questions similar to the following:

I see a jumper in a shop window priced at £45. The next day the jumper is reduced in price by 30%. How much does the jumper cost now?

  • A child would need to work out 10% of £45 (£4.50) and then multiply this by 3 (£13.50).
  • They would then need to take this £13.50 away from the £45, which would give them £31.50.

Children may also need to compare fractions of amounts to percentages of amounts, for example:

There are 140 sweets in a jar in a sweet shop. Katy buys 2/5 of the sweets. John buys 45% of the sweets. Work out how many sweets each child buys. Who buys more sweets?

  • To work out what Katy buys, children would need to find 1/5 of 140 which is 140 ÷ 5 = 28.  2/5 would therefore be 28 x 2 = 56.
  • To work out what John buys, children would need to find 10% of 140, which is 140 ÷ 10 = 14.
  • They would then need to multiply this by 4 to make 40% which would equal 56.
  • To find the 5% they would need to halve 10% of 140 (14) which would make 7.
  • Then to find 45% they would need to add the 40% (56) to the 5% (7) which would make 63.
  • So Katy buys 56 and John buys 63 sweets, which means John has bought more.