Important update from TheSchoolRun
For the past 13 years, TheSchoolRun has been run by a small team of mums working from home, dedicated to providing quality educational resources to primary school parents. Unfortunately, rising supplier costs and falling revenue have made it impossible for us to continue operating, and we’ve had to make the difficult decision to close. The good news: We’ve arranged for another educational provider to take over many of our resources. These will be hosted on a new portal, where the content will be updated and expanded to support your child’s learning.
What this means for subscribers:
- Your subscription is still active, and for now, you can keep using the website as normal — just log in with your usual details to access all our articles and resources*.
- In a few months, all resources will move to the new portal. You’ll continue to have access there until your subscription ends. We’ll send you full details nearer the time.
- As a thank you for your support, we’ll also be sending you 16 primary school eBooks (worth £108.84) to download and keep.
A few changes to be aware of:
- The Learning Journey weekly email has ended, but your child’s plan will still be updated on your dashboard each Monday. Just log in to see the recommended worksheets.
- The 11+ weekly emails have now ended. We sent you all the remaining emails in the series at the end of March — please check your inbox (and spam folder) if you haven’t seen them. You can also follow the full programme here: 11+ Learning Journey.
If you have any questions, please contact us at [email protected]. Thank you for being part of our journey it’s been a privilege to support your family’s learning.
*If you need to reset your password, it will still work as usual. Please check your spam folder if the reset email doesn’t appear in your inbox.
What is place value?
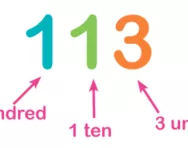
What is place value?
How are children taught to understand place value in KS1?
In school two maths aids are used to help make place value clear to children.
Dienes blocks are blocks in which cubes represent ones, rods of ten cubes represent tens, flats of 100 cubes represent hundreds and blocks of 1000 cubes represent thousands: 
In Key Stage 1, a child might be given some ten and ones. Dienes blocks and asked to make a number such as 43. They would need to select 4 tens rods and 3 ones blocks. This makes it very clear to them that a two-digit number it made up of tens and ones. It also helps them to practise counting in tens.
Arrow cards look like this:
A child might be asked to make the number 34 using arrow cards. They would need to take the 30 and the 4 and put them together so that the arrows were lined up. This again helps to make clear that a two-digit number is made up of tens and ones.
It is absolutely vital that children understand place value before they can go onto adding and subtracting two-digit numbers.
Place value in KS2
In Year 3, children might be given Deines blocks and arrow cards to help them with adding a pair of two-digit numbers. This would enable them to partition the numbers, so that they could add the tens first and then the ones. A Year 3 child would also be expected to know the place value of digits in three-digit numbers.
In Year 3, children also need to know what happens to a number when it is multiplied by 10 or 100. It is really important here that they are aware that the number moves to the left, rather than talking about 'adding zeros' (when children move onto dividing by 10 to find a decimal answer, they will not be able to use the strategy of adding or removing zeros).
This is a common method used by teachers to show children how to multiply by ten:
When multiplying 6 by ten, the number moves to the left and a zero is put in, so the number becomes 60.
If you multiply 6 by 100, the number moves two places to the left and zeros are put in, so the number would become 600.
In Year 4, children would need to use their knowledge of place value to work out sums and difference of pairs of multiples of 10, 100 or 1000. They might be asked to mentally work out 80 + 40 in which case they would need to 'cross 100' to find the answer 120. They might be asked to work out 700 + 600 or 8000 + 3000, in which case they would be crossing 1000 and 10,000. A number line can be helpful in these instances.
Year 4 children also need to start to understand the place value of decimals. A blank hundred square is a good way of demonstrating this:
With the above two decimals, you can make it clear that 0.41 is 41/100 and 0.02 is 2/100.
Sometimes teachers use small whiteboards marked like this:
onto which children have to write a number that the teacher has said out loud. For example: the child might be asked to write the number 5.72. They might then be asked to add 0.1 to this number, at which point they would need to change the number to 5.82. They might be asked to subtract 0.01, at which point they would need to change the number to 5.81.
In Year 4 children need to learn how to divide numbers by 10 and 100. Teachers usually teach this using this method:
When dividing 6 by ten, the number moves one place to the right and becomes 0.6.
If you divide 6 by 100, the number moves two places to the right and becomes 0.06.
In Year 5, children need to use their knowledge of place value to work out sums and differences of decimals. For example, they would need to know that adding 0.8 and 0.4 results in 'crossing 1' because the answer is 1.2. A number line can be helpful in demonstrating this:
In Year 6, children need to know their knowledge of place value to work out multiplication and division of decimals. For example, when working out 0.6 x 4, they would need to know that this is the same as working out 6 x 4 = 24, but then the answer needs to be divided by 10 (because 6 divided by 10 is 0.6) to make the answer 2.4.