What is 'shared between'?
What is 'shared between'?
'Shared between' is a term used in word problems that involve division. An example of such a Key Stage 1 problem might be:
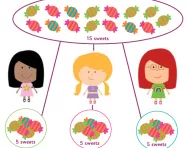
Nathan has 20 sweets. He shares them equally between his four friends. How many sweets does each friend get?
Teachers will often give children counters to work out these problems. They may encourage them to draw stick men to represent the four friends, and then show them how to share the counters equally between the four friends, by giving one to each child until there are none left.
'Sharing' division word problems in primary school
In Year 1, children need to share into groups of 2, 5 or 10 (this coincides with their learning of the 2, 5 and 10 times tables in Key Stage 1).
In Year 2, children would be introduced to various symbols including ÷ and would need to know that this symbol means 'divide' or 'share'.
In Year 3, children may still come across the word 'share' and will be moving onto dividing larger numbers by one-digit numbers, such as 50 ÷ 4.
As children get more confident with their times tables and start to learn how these are related to division, children (especially in Year 3) are encouraged to use the inverse to work out problems like the above one. So instead of sharing 20 counters into 4 equal groups, they realise that the above problem would be written as a number sentence like this:
20 ÷ 4 =
They would then use their knowledge of times tables and the inverse to remember that 4 x 5 = 20, therefore the answer must be 5.
Children in later Key Stage 2 will still come across the word 'share' in their maths problems. In Year 6 children need to be able to mentally divide a decimal by a unit. For example in the following problem:
I have 1.2 litres of orange juice. I need to share this equally between six people. How much orange juice will each child get?
- One way of doing this is to convert the 1.2 litres into 1200ml and then recall that there are 5 lots of 200 in 1000, so there must be six lots of 200 in 1200, therefore each child will get 200ml.
- Another way of doing this is to think of the question as a number sentence: 1.2 ÷ 6 =
- Since a child would know that 12 ÷ 6 is 2, they could then work out that 1.2 ÷ 6 = 0.2, so give their answer as 0.2 litres or 200ml.
Children at this stage should also have efficient methods for dividing larger numbers. For example, they may come across a problem as follows:
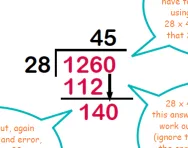
John has 362 marbles in a jar. He shares them between 7 people. How many marbles does each friend get? How many marbles are left over?
Here a child could use the bus stop method:
Or they might choose to think about what they would multiply 7 by to make a number close to 362. They might work out that 5 x 7 = 35, therefore 5 x 71 = 351 with 5 left over, therefore each child would get 51 marbles with 5 left over.
Give your child a headstart
- FREE articles & expert information
- FREE resources & activities
- FREE homework help
