✕

Important update from TheSchoolRun

For the past 13 years, TheSchoolRun has been run by a small team of mums working from home, dedicated to providing quality educational resources to primary school parents. Unfortunately, rising supplier costs and falling revenue have made it impossible for us to continue operating, and we’ve had to make the difficult decision to close. The good news: We’ve arranged for another educational provider to take over many of our resources. These will be hosted on a new portal, where the content will be updated and expanded to support your child’s learning.

What this means for subscribers:

  • Your subscription is still active, and for now, you can keep using the website as normal — just log in with your usual details to access all our articles and resources*.
  • In a few months, all resources will move to the new portal. You’ll continue to have access there until your subscription ends. We’ll send you full details nearer the time.
  • As a thank you for your support, we’ll also be sending you 16 primary school eBooks (worth £108.84) to download and keep.

A few changes to be aware of:

  • The Learning Journey weekly email has ended, but your child’s plan will still be updated on your dashboard each Monday. Just log in to see the recommended worksheets.
  • The 11+ weekly emails have now ended. We sent you all the remaining emails in the series at the end of March — please check your inbox (and spam folder) if you haven’t seen them. You can also follow the full programme here: 11+ Learning Journey.

If you have any questions, please contact us at [email protected]. Thank you for being part of our journey it’s been a privilege to support your family’s learning.

*If you need to reset your password, it will still work as usual. Please check your spam folder if the reset email doesn’t appear in your inbox.

What is symmetry?

What is symmetry?
We explain what symmetry is and how primary-school children are taught to find the line of symmetry and draw lines of symmetry on different shapes.

What is symmetry?

Something is symmetrical when it is the same on both sides. A shape has symmetry if a central dividing line (a mirror line) can be drawn on it, to show that both sides of the shape are exactly the same.

Learning about symmetry in primary school

Children start to learn about symmetry in Year 2, where they might be given the following shapes and asked to draw lines of symmetry on them. Often, it is a good idea for children to be given cut out shapes which they can fold in half, so that they can be really clear that one side is the same as the other:

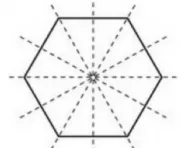
In Year 4, children are asked to identify lines of symmetry in 2D shapes presented in different orientations. They will need to become aware that shapes have more than one line of symmetry. They may be asked to look at these regular shapes and think about how many lines of symmetry they can find. Again, it is helpful for them to cut out the shapes and fold them in half, then look at how many folds they have made.


They might also be asked to classify shapes according to various properties, including line symmetry. They may be given a group of shapes and asked to put them into a Carroll diagram as follows:

Children in Year 5 begin to reflect shapes in a mirror line. They may be asked to complete the shading in a shape after reflecting it in a mirror line, or be given half a shape with a mirror line and asked to draw the other half. The shape is usually given on squared paper so that they are able to complete the shape accurately:

In Year 6 children will be asked to draw shapes on the co-ordinate plane and then reflect them in the axes so that they appear in all four quadrants (see below).

Reflecting shapes in a mirror line

Children will also be given a quarter of a shape and then asked to reflect it in two mirror lines, for example:
  

Alternatively, they may be given a shape with shaded squares reflected in two mirror lines and asked to complete the shading of the squares:
  

In Year 6, children may be given a shape on some squared paper and asked to reflect the whole shape in two mirror lines. For example:
  

Sometimes symmetry is combined with coordinates. A child may be shown a shape as follows and then asked to reflect it in the mirror line and then write down the co-ordinates of 'A' on the reflected shape (in the example below the reflected A vertex would have the coordinates 3, 7).